博文
My experience of using Fourier transformation (我使用傅利叶变换的经历)
||
I was shocked the first time when I knew Fourier transformtion. It is amazing that nearly any function could be expanded as a sum of simple sine and cosine functions. It is very different from another famous expansion, Taylor expansion.
Taylor expansion focuses on one point while Fourier expansion cares about the global feature.
Fourier transformation has many applications in mathematics and physics. In some cases, a difficult problem could be easy after Fourier transformation. This is the trick.
I have two pieces of experiences of using Fourier transformation to obtain the probability distribution function (PDF) of a random variable.
In 2018, I tried to understand the working mechanism of the boosted decision tree (BDT) algorithm. I assumed that all trees are weak learner and attempted to obtain the PDF of the final BDT score.
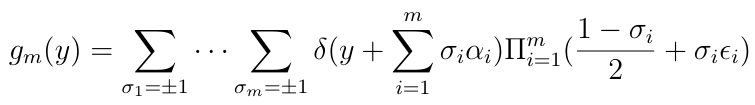
As shown in the equation above, I wanted to know the distribution of gm(y) and it was difficult at first glance. But after applying a Fourier transformation, the problem turned out to be very clear and easy to handle. More details could be found in this arXiv ( https://arxiv.org/pdf/1811.04822.pdf ).
The second experience happened the other day. I tried to improve a set of asymptotic formulae to describe the distribution of a test statistic used in the data analysis in particle physics experiments. To be exact, I want to know the PDF of mu_hat as shown below, where n_i abides by a Poisson distribution.
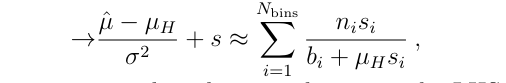
It was also difficult, but I thought Fourier transformation would work one second later. Indeed, I can easily find a good approximation of the PDF of mu_hat using the Fourier transformation trick. More details could be found in this arXiv ( https://arxiv.org/pdf/2101.06944.pdf ).
I feel lucky when I can really apply the mathematics I have learnt to the field where I am working. It is ofcourse not expected when I learnt mathematics.
https://wap.sciencenet.cn/blog-3401446-1267896.html
上一篇:First observation of Mercury 第一次看到水星
下一篇:A problem about the AM-GM inequality (一道关于均值不等式的题目)